CV: Erynn Sangster
Personal Statement
Personal Profile
I am an enthusiastic individual who supports the idea that Primary School Pupils need an exciting classroom teacher to help them with their development of English.I am currently at Dundee University studying MA (Hons) Primary Education. I have passed and finished my first year and during it I experience 6 weeks of placement in a P4 classroom where I had full responsibility for lessons, safety and organisation. I have accumulated high teamwork, communication, patience, reliability and responsibility skills through my education, extra-curricular activities and work experience.
Skills
Communication/Teamwork
- Through my experience in being a dancer/choreographer I use this skill to instruct and work with other dancers effectively.
- My previous part-time jobs involved using this skill to deal with customers and to work along side other employees smoothly.
Thinking
- I have great thinking skills to find solutions to problems, I used this a lot during my previous jobs if we would get customer complaints or questions.
- In my school placements and dancing I am able to come up with new ideas and to be creative to make the lessons more engaging.
Organisation
- I built up my time management skills when doing lessons so I would not run over or under the allocated time. Also making sure I stick to deadlines set by the university.
- Making sure my lesson plans matched up and followed the experiences and outcomes of the Curriculum for Excellence.
Interpersonal
- I approach things in a positive way and I have enthusiasm for working with children.
- Promote positive behaviour in the classroom through praise and rewards and use sanctions for poor behaviour to encourage/remind the pupils of the rules and expectations.
Education
Kemnay Academy August 2010 – June 2016
National 5: Business Management (A), German (A), Maths (B), English (A), Art & Design (A), Biology (B).
Higher: English (B), Art & Design (A), Biology (C), Business (A), Drama (C).
Advanced Higher: Art and Design (Expressive) (B)
University of Dundee September 2016 – 2020
MA (Hons) Education
Education modules have included, teaching across the curriculum, values, pedagogical, discovering mathematics, languages and educational studies.
Work Experience
Sales Floor Customer Assistant August 2014 – December 2014
Marks & Spencer
Customer Assistant February 2015 – September 2016
Michael Howden
Co-operative
Communication and teamwork skills were used when working with customers.
Primary School Student Teacher March 2017 – May 2017
Alison Macgregor
University Primary School Placement
During my 6 week period of working at the school I had a lot of responsibility over children to uphold while working alongside the classroom teacher. I had to plan lessons for each day and make sure I was organised with resources for each of them. I had the responsibility whilst planning lesson to make sure my l time management was correct and making sure each child was getting the help they needed, for the care and well-being of each child and supporting each individual with their needs.
Nursery Assistant June 2017 – August 2017
Rachel Farman
Wee Rascals Summers Nursery
I practised my discipline and praising skills along with experiencing very early development of reading, writing, talking and listening skills from the children. I worked alongside a couple other assistants in each room and worked with children ranging from 6 months to 5 years old.
Interests & Achievements
I do dance and lacrosse at university; these sport support teamwork and communication.
Costa Rica Outlook Expedition June 2015
A 3-week expedition to Costa Rica with a team from my school. Whilst there we were in charge of our food, travel/accommodation and money. It was up to us to get us through these 3 weeks. We had 3 phases, a turtles project, trekking and rest/relaxation. It really tested our teamwork skills and made us all more independent as individuals.
Prefect & House Captain August 2015
I was a prefect at Kemnay Academy. This involves setting examples to younger years and keeping the school grounds in order. Managing queues at lunch and break and presenting ones self as a responsible citizen. Being as House Captain made us work as a house team to earn points and be enthusiastic towards the younger years.
Dance Leadership Level 4 November 2015
Grade 5 Saxophone March 2014
Grade 5 Tap May 2016
Rock Challenge February 2015


 To make something louder you need to add more energy to it, the term amplitude can be used to refer to loudness. Amplitude is the maximum height of the wave from its resting position – the greater the amplitude, the louder the sound. Pitch is to do with the frequency or number of vibrations per second. Frequency is measured in hertz (Hz). The closer together the waves are and the higher the pitch. On a guitar sting, the shorter the sting the higher the pitch will be as the vibration have less material to cover therefore the pattern of oscillations is more regular.
To make something louder you need to add more energy to it, the term amplitude can be used to refer to loudness. Amplitude is the maximum height of the wave from its resting position – the greater the amplitude, the louder the sound. Pitch is to do with the frequency or number of vibrations per second. Frequency is measured in hertz (Hz). The closer together the waves are and the higher the pitch. On a guitar sting, the shorter the sting the higher the pitch will be as the vibration have less material to cover therefore the pattern of oscillations is more regular. Once I came across Liping Ma’s book and read up about her theory then I was able to understand it better and see the links in all my future lectures. Ma wanted to understand why the U.S.A were in a much lower rank for test results than China was. Ma (2010) concluded that the reason the U.S.A were so behind was because teachers didn’t obtain an extensive understanding of elementary mathematics. She figured that during a teachers training they should be made aware and become habitual with basic (fundamental) mathematics as this is what the teachers in China have knowledge on from the start (Ma, 2010).
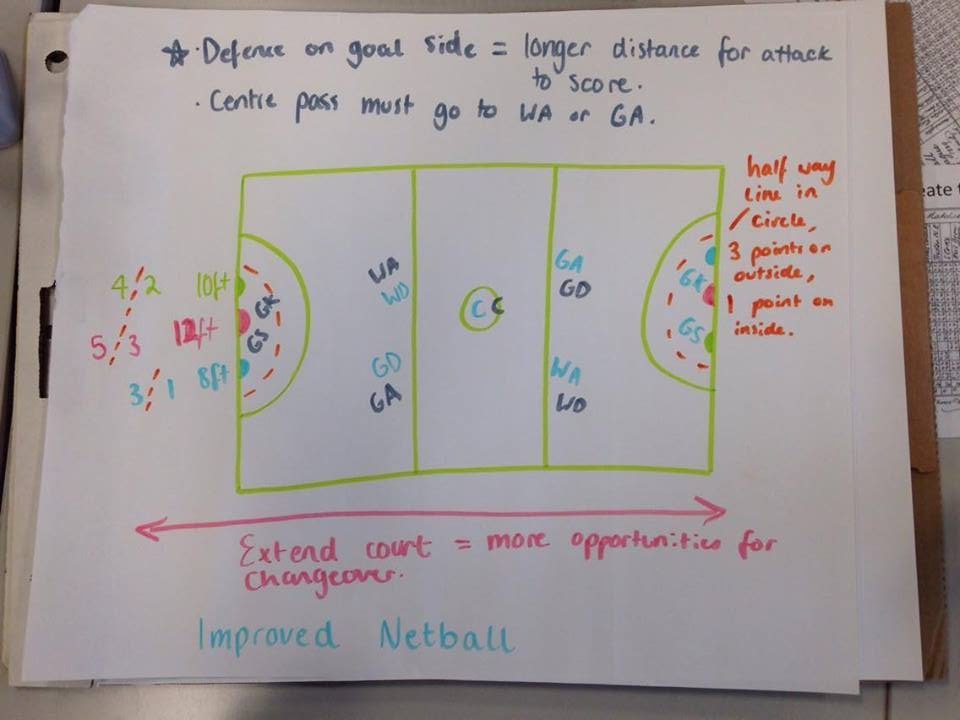
Once I came across Liping Ma’s book and read up about her theory then I was able to understand it better and see the links in all my future lectures. Ma wanted to understand why the U.S.A were in a much lower rank for test results than China was. Ma (2010) concluded that the reason the U.S.A were so behind was because teachers didn’t obtain an extensive understanding of elementary mathematics. She figured that during a teachers training they should be made aware and become habitual with basic (fundamental) mathematics as this is what the teachers in China have knowledge on from the start (Ma, 2010). Firstly in the lecture, Richard had us look at an old football score table for 1888-1889 and compare it to the ones we see today. We looked at the rankings, the wins, loses and draws and how many the won for and lost against. To try and make sense of the old table we redesigned it by firstly putting the football team names in order from rank 1-12 and followed that with their point beside them. By ordering the team we used the fundamental mathematics category, from Liping Ma, of basic mathematical principles (Ma, 2010). We used counting to help us order and matching to match up the points form the old table to then new order of teams.
Firstly in the lecture, Richard had us look at an old football score table for 1888-1889 and compare it to the ones we see today. We looked at the rankings, the wins, loses and draws and how many the won for and lost against. To try and make sense of the old table we redesigned it by firstly putting the football team names in order from rank 1-12 and followed that with their point beside them. By ordering the team we used the fundamental mathematics category, from Liping Ma, of basic mathematical principles (Ma, 2010). We used counting to help us order and matching to match up the points form the old table to then new order of teams.