Category Archives: 2 Prof. Knowledge & Understanding

A Victorian for a Day

“Make sure you remember to put that scarf on!” shouted my mum as I left her to start the walk into the school playground, “You won’t look like a Victorian if you don’t put it around you like a shawl!”. Months of hard work and dedication had led to this day, the day where we finally got to showcase all of our work on the Victorians to our parents and carers. My whole family was involved by this stage – my gran had sown an outfit together for me, my mum had taken time off work to come and see our presentation, my dad had raided the garage to find parts for the Victorian house we made as a group – so there was a lot riding on this to go well. I could not wait to showcase all of our hard work we had put into creating our props and speech for the presentation – our Victorian house we made from a show box, our letters written from the perspective of a Victorian man or lady, our poster of all the inventors we had learnt about from the era, and the rest. We had even transformed the classroom into a class from the Victorian times so you were transported into the era as soon as you walked through the door – it was so realistic!

I remember this day in Primary 7 so vividly as the sharing of our learning to parents and carers was made into such a big deal that it was difficult not to get excited about it. I recall being so happy to be able to share my learning with my family that it made me more motivated to learn as much as I could in the lead up to the shared afternoon.

My teacher was super enthusiastic about the topic and really made it come to life through the stories she told us, the case studies she shared and the transforming of the classroom. We even had to sit in rows one day and call our teacher ‘Miss’ – it felt as if I had been transported back in time as everyone played their part in creating the context. I even recall our Head Teacher getting involved and pretending to be really really strict! The context of the learning was so engaging and stimulating that it became something that I looked forward to learning about everyday.

My teacher would also present problems to us from different characters or real life people we had been learning about such as Ebenezer Scrooge. One of the challenges I recall was to create a timeline of events during Queen Victoria’s reign. Before we did this, we decided as a class that it would be best to create timelines of our own lives first so that we understood how to apply it to a context such as the Victorians. Because the teacher let us lead the learning during most of the topic, it felt as if we had responsibility over what we were learning, thus making it more relevant and engaging for us as a class. She was also extremely flexible in letting us direct the learning as she would encourage the class to investigate questions they had and supported them in doing so.

Overall, I feel that this experience was so memorable for me as it gave me the opportunity to share and develop my knowledge and understanding with my family, direct the learning taking place, and be fully emerged in the learning through the engaging context that my teacher created.

The Beauty of Mathematics

Leave a reply

CAN MATHEMATICS BE BEAUTIFUL?

If you take a look inside a child’s math jotter you will most likely find some numbers written on parallel lines to form equations and answers. But, is it possible to use math to form or create something which is not numbers based? Can we create something more exciting and creative other than stereotypically dull equations found in a child’s maths textbook?

Using Maths to Enhance Art

To my surprise, we can! We can actually use maths to ENHANCE and IMPROVE our abilities within the creative arts sector. Barrow (2014) suggests that maths and art are subjects which can be seen as coming in ‘hand in hand’ with one another. He also emphasises that patterns – which are the fundamental basis of art – are all based upon mathematical concepts (Barrow, 2014), such as symmetry, angles, rotation, shape etc – what Ma (2010) describes as the basic ideas of a fundamental understanding of maths. Buck (2011) agrees with this, saying that the patterns that artists create all have their individual algorithms, a mathematical set of instructions, which they must follow in order to correctly create the pattern.

Rule of Thirds

Even photographers can use maths to enhance their ability to capture aesthetically pleasing images. By splitting their lens into nine equal squares/rectangles, they can position the main subject of their photo along the intersecting lines of the squares. This opens up space behind or around the subject which the eye is then drawn to, meaning there is more than just the subject of the photo that is attractive to the eye at first glance.

Image 1 – Rule of Thirds

Self-Portraits

We can also use maths to transform ourselves into expert portrait drawers (ish)! By implementing basic concepts of shape and fractions into a portrait piece, we can enhance the end product. Using these concepts increase the likelihood of the features of the face being proportionate to each other, and therefore, making it more realistic than a piece that has not used maths. These basic concepts can be implemented when portrait drawing due to the symmetrical nature of the face, which serves as a source of beauty in itself (Zaidel and Hessmian, 2010).

For example, through splitting the outline of the face into quarters we can correctly identify where each of the facial features should be placed in respect of each other. Using maths, we can work out that the eyes should be positioned half way up the face and a fifth of the width of the face in from the outline (See picture below).

Image 2 – Using maths to sketch a face

I attempted to use this technique to draw a portrait of a face. Here is the before and after…

It really amazed me how different and how much better my second attempt at drawing a face was using just a little bit of maths! Being someone whose art ability is incredibility limited, it was surprising how easy the maths made drawing an in proportion and to scale face. Thus, this small adjustment to the way I approached this task, in my opinion, increased the aesthetic nature of my work.

Using Maths to Create Art

But it doesn’t just stop there! Maths can also be beautiful when used in solitude, not in conjunction with other subject areas – we can use maths alone to CREATE art.

Curves of Pursuit

By repeating a shape at the same interval multiple times, we can create even more shapes and beautiful patterns.

For example, using the square. By drawing a square and then drawing another square within it by measuring 1cm away from the right of each of the corners and then jointing these points up, we can create another square within it. When you repeat this action, you end up with something like this…

As you can see, I have ended up with a spiral motion within the original square, as well as individual triangles running through the curves. This type of mathematical phenomenon demonstrates how using one simple shape can create a very dynamic new image which is very pleasant to look at. I think that is amazing that we can use maths to create such intricate patterns, all from using a simple shape.

From my research into maths and art, I now understand the importance of being able to apply mathematical knowledge and skills to wider areas of society. I will no longer view maths as an isolated discipline of which numbers are the underlying fundamental basic idea (Ma, 2010). I hope to gain a better understanding of how maths can influence our society (other than in the creative arts) by exploring other sectors which it is intrinsically connected with, such as science and engineering.

In conclusion, the end result of mathematics does just have to include formulae, numbers and equations. It may result in beautiful aesthetics such as patterns, painting, images, photographs, tiling and much more. We can use maths to enhance our ability within the arts such as through using fractions and shapes in portrait drawing, we can increase how realistic the end drawing looks. You can also use maths alone to create art. Again using the basic idea of shape, Ma (2010), we can create beautifully aesthetic patterns from just one simple shape, for example, in curves of pursuit images.

References

Barrow, J.D. (2014). 100 Essential Things You Didn’t Know You Didn’t Know About Maths and the Arts. London: The Bodley Head. Available at: https://www.vlebooks.com/vleweb/Product/Index/673669?page=0 [Accessed 2 November 2018].

Buck, G. (2001). MATHEMATICS AND ART: Algorithms of Boundless Beauty. Science, 292(5516), pp.445-446.

Ma, L (2010) Knowing and Teaching Elementary Mathematics. Oxon: Routledge.

Zaidel, D. and Hessamian, M. (2010). Asymmetry and Symmetry in the Beauty of Human Faces. Symmetry, 2(1), pp.136-149.

Image 1 – Rule of thirds applied on Mädchen am Strand (2015) Wikipedia. Available at: https://commons.wikimedia.org/wiki/File:Rule_of_thirds_applied_on_M%C3%A4dchen_am_Strand.jpg [Accessed 2 November 2018].

Image 2 – Maths careers. Available at: http://www.mathscareers.org.uk/article/figure-drawing/ [Accessed 2 November 2018].

Space is big, really REALLY big

Leave a reply

One of the ways that teachers across Scotland are trying to encourage positive affective domains within children in their maths is by making it more relevant.

By applying mathematical concepts to everyday real life concepts, we can encourage children to appreciate the application of maths in society. For example, as teachers we cover a range of inter-disciplinary topics as part of the Curriculum for Excellence (Scottish Government, 2008), which often allows them to apply mathematical concepts that they have been taught to real life scenarios. However, do we allow our children to recognise the importance of these topics, rather than them seeing it as just another area which they need to learn?

One of these topics – a topic familiar with every child – is Space. My understanding of space at Primary School was that we had a sun and a moon and 7 other planets surrounding us in an area known as our solar system. However, is this where our knowledge of space should end? At the edge of our solar system? Could we not explore our universe with them even further by delving deeper into the whole new worlds which surround our solar system, allowing children to recognise the incredibly vast space that we live in. Thus, I ask myself…

Could maths give us a better understanding of the depth of our universe?

Early thoughts…

Greek astronomers such as Ptolemy believed that our solar system was geocentric – that the earth was in the centre of our solar system and the other planets orbited around it. He also believed that the planets were an equal distance away from one another. As he was unable to use experimentation to prove his ideas, he tailored his maths to fit them and went on to make everyone believe his predictions through his use of mathematics (Falkner, 2011).

Image 1 – Geocentric model

Ptolemy used maths to gain a better understanding of our universe, but yet his knowledge of it was not correct. He showed how maths can be adapted to fit our beliefs, but it may not give us an understanding of the underlying reality.

However, his maths would have given him a greater understanding of the universe if he had conducted experiments in regards to the distances between the planets. He then could have went on to use maths to confirm his experiment results.

Another Greek astronomer Copernicus did exactly this. He used constant and more in depth observations than Ptolemy to figure out that we have a ‘heliocentric system’ (where the sun is at the centre of the solar system) and that the planets are unequal distances apart. Through this, he was then able to use maths to prove his theory to the public (Falkner, 2011).

So how can we use the maths and the theories that Copernicus created to give us a better understanding of our universe?

I was challenged to recreate our current solar system as we know it using the distances each planet is from the sun, and here is how it turned out (Apologies for the poor quality)…

Image 2 – Planets in our solar system distance from the sun

*Please note that the planets are not to scale with the toilet roll, although they are to scale with each other.

It took me approximately 30 minutes to devise a scale for my planets to sit on due to the HUGE differences in distances that the planets are from the sun. The first four planets were all between 57-230 million km away from the sun whereas the furthest away planet, Neptune, was over 78 times further away from the sun than the nearest planet Mercury! This made it incredibly difficult to devise a scale which would clearly show the difference in distances between the planets and the sun.

Upon finally deciding that I would use 500 million km per toilet ply as a scale for my solar system, I was finally able to align my planets! Mercury, venus, mars and earth all appear incredibly close to one another, when in actual fact they are at least 50 million km away from each other. If it wasn’t for the maths underlying my model I would have assumed that the planets are actually pretty close together. The maths involved in this tells me that actually the pictures we were shown in primary of planets being perfectly aligned away from the sun were in fact, incorrect. (See an example of these types of images below.)

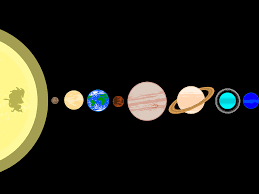Image 3

If you then consider other entities within our milky way you can see how small we really are in this vast world. We are just one of hundreds of thousands of solar systems that make up the milky way (Reynolds, 2018).

If the diameter of our solar system is around 250 billion km, then can you imagine the enormity of our milky way. But it doesn’t just stop there. There are 100-1000 billion galaxies in our universe. IC 1101 is the biggest, at 11,504,106,950,000,000,000 km (Creighton, 2016).

Implementing this in the classroom

It is these numbers that help us to gain a better understanding of the vast universe that we live in. Basic ideas can be explored using the maths that Copernicus created, such as scale, shape and area. These basic ideas are what Ma (2010) describes as the fundamental principles of mathematics. This suggests that by developing children’s spatial awareness further when exploring the topic of space, we are also helping them to become more competent in their maths skills. It is these skills that would allow them to recognise a wrongly scaled solar system if they ever saw one!

They could then go on to implement these skills in other areas of the curriculum such as art and design technology. For example, using shapes and scale to draw a correctly proportioned human face (see next post). These interconnections between their areas of learning is something that Ma (2010) suggests is key is allowing children to see the relevance and importance in what they are being taught.

In conclusion, Copernicus’ theories surrounding the scale of our solar system allows us to gain a better understanding of the magnitude of our universe. The magnitude of the numbers he devised to prove his theory gives us an indication of just how small we actually are in this world. Using the numbers he came up with, we can create a correctly scaled replica of our solar system and other bodies in the universe to gain further knowledge and understanding of the world we live in. Children can use the math surrounding his theories to develop the basic ideas of shape and scale, whilst being able to acknowledge that their is more to the universe than just our solar system!!

References

Creighton, J. (2016) The Largest Galaxy In the Known Universe: IC 1101. Available at: https://futurism.com/ic-1101-the-largest-galaxy-ever-found [Accessed 25 October 2018.

Falkner, D.E. (2011) The Mythology of the Night Sky: An Amateur Astronomer’s Guide to the Ancient Greek and Roman Legends. Springer Science+Business Media: London.

Ma, L (2010) Knowing and Teaching Elementary Mathematics. Oxon: Routledge.

Reynolds. S. (2018) Maths in Astronomy [PowerPoint Presentation]. ED21006: Discovering Maths. University of Dundee.

The Scottish Government (2008) The Curriculum for Excellence: Building the Curriculum 3. Edinburgh. Available at: https://www.gov.scot/resource/doc/226155/0061245.pdf [Accessed 23 October 2018].

Image 1 – Bfritz (2013) Available at: https://www.proprofs.com/quiz-school/story.php?title=history-astronomy_1 [Accessed 25 October 2018].

Image 3 – Pixabay (2017) Available at: https://pixabay.com/en/solar-system-space-planets-order-2453896/ [Accessed 25 October 2018].

What do you mean numbers don’t exist?!

1 Reply

Yes, you did see it right. Apparently, numbers do not exist. My mind is still trying to process how such a concept could even be possible after believing all my life that numbers are the fundamental basis of mathematics. After much time pondering and challenging this, it appears to be true…

We use numbers everyday, from the numerals on our watches, the numbers on our calendars to the sizing on our clothing. Each of these numbers we use represent something, but what if we substituted our common base-ten numbers for something different? Would we still be able to count, tell the time, use money etc?

Collins (1998) suggests that by taking a nominal view of numbers, we can. He suggests that we can use any number system in the world (or a completely new one if we wished) to represent a size and it would still make sense, as long as we knew what each symbol represented. Society has constructed these notions that 1 is less than 4, and 4 is 3 more than 1 etc. However, Collins (1998) argues that we could equally use the symbol ‘4’ to represent one item and the symbol ‘1’ to represent four items, thus showing the flexibility of numbers as we know it. This is something I would never have considered as I thought the number system was a rigid structure, something which could never be changed. Nominalists see numbers as ‘fictions’ – symbols which aid and support our understanding of mathematics and everyday life, meaning we could use other symbols or entities to do the exact same job. The fact that we use different number systems around the world, such as octal, mayan and Arabic, supports this theory, and suggests that numbers are something which we have been predisposed to use through the work of many mathematicians which have came before us.

On the other hand, Collins (1998) explores the view that many realists take. We use numbers everyday in order to make sense of, and complete a wide variety of tasks everyday and thus, numbers must be real if we use them. Fine (2009) suggests the links that we have formed between numbers and the properties we have given them suggests that their existence must be true. For example, we have developed the concept of ‘prime’ numbers within our number system. Although, is this not another made up concept used to help us understand and make links within our made up number system? Confusing, isn’t it!

We could have used the word prime to represent another property of numbers, such as those divisible by 2, or every number ending in 1. Thus, this could be applied to other items we represent using language in our everyday lives. We could use the word ‘table’ to represent a ‘chair’ or vice versa, as long as we had an agreed understanding as a society that a ‘table’ actually represented what we know today to be chair.

Randy Palisoc develops this idea is his ted talk (see video below) by suggesting that numbers are a language we use to make sense of the world around us (TEDx Talks, 2014), just like we have constructed the letter system (the alphabet) and thus words to be able to communicate with one another.

Overall, I believe that the debate on the existence of numbers is an incredibly interesting one. I acknowledge that we use numbers everyday to perform basic functions, and thus they must be real as such. I also share Collin’s (1998) nominalist view that we could substitute other symbols for our base-ten number system and would still be able to perform basic arithmetic and daily tasks involving what our numbers represent, whether that be time, quantity of money or clothes sizes – the list goes on. The language we use for numbers, whatever this may be, is one which we will most likely always require to be a fully functioning member of society. Through this, is it clear to see that is imperative that we have a number system in place which allows us to represent different quantities and sizes etc, even if it is made up!

References

Collins, A.W. (1998) On the Question ‘Do Numbers Exist?’, The Philosophical Quarterly, 48 (190), pp. 23-36. Available at: https://www.jstor.org/stable/2660370?seq=1#page_scan_tab_contents [Accessed 8 October 2018].

Fine, K (2009) The question of ontology, Metametaphysics: New Essays on the Foundations of Ontology, pp. 157-177. Available at: https://philpapers.org/rec/FINTQO-2 [Accessed 8 October 2018].

Image – Wikipedia (2018) Numeral Systems of the World. Available at: https://commons.wikimedia.org/wiki/File:Numeral_Systems_of_the_World.svg [Accessed 8 October 2018].

Video – TEDx Talks (2014) Math isn’t hard, it’s a language | Randy Palisoc | TEDxManhattanBeach. Available at: https://www.youtube.com/watch?v=V6yixyiJcos [Accessed 8 October 2018].

Maths IS important!

Leave a reply

“Is maths important?” my lecturer asked during a recent Discovering Mathematics workshop. My exam driven former self would have explicitly answered no to this question, as I had all sorts of weird and wonderful equations and rules drilled into my head at this point – none of which I have actually implemented within my everyday life, but hey! At least I passed my exam…

As I am no longer in this environment, and have begun my journey back into the
Primary School setting, I have began to re-establish the view that maths IS important. But what caused my shift in opinion on the importance of maths when I reached High School?

I believe that in Primary School we are taught in such a way that allows us to have a conceptual understanding (the HOW and WHY) of the area we are learning, formally known as relative understanding (Skemp, 1989). Whereas in High School, my experience was being taught the ‘HOW’ of a branch of mathematics – known as a procedural or instrumental understanding(Skemp, 1989). Upon asking my Higher maths teacher why we needed to know the likes of the quadratic formula, the cosine rules and how to differentiate an equation, he replied “you don’t – you just need to know HOW to do it”. The fact that my own maths teacher was teaching us based on instrumental understanding may suggest that either; he underestimated the power of understanding the WHY, or he was not required to teach it, so he simply didn’t. As long as we managed to get the answers right, even if we did not understand why it was right, he would say we were doing a good job and were heading on route to pass the exam.

Not knowing the WHY behind the mathematics I was being taught made it seem irrelevant and useless to me, hence why I refused to see its importance at the time. Ma (2010) suggests that having a profound (or relative) understanding of mathematics enables learners to see the ‘interconnectedness’ between concepts, thus they are able to apply their knowledge to a wider range of scenarios than having an instrumental understanding alone. His theory dismisses teaching towards an procedural understanding (like my teacher did), as it restricts the wider connections an individual can make between what they are learning and other areas of the curriculum, and also in their everyday life (depending on the nature of the topic being explored).

My belief that High Schools are teaching towards the objective of passing exams is strengthened by the data released by the Scottish Government (2012), as it shows the percentage of pupils performing very well at the level they are working at in maths decreases by 18% from Primary 6 to S2 (see graph below). This statistic, although not surprising to me, is a shocking reminder that the strategies for teaching maths in High Schools is not as effective as those demonstrated within Primary School.

Overall, I believe that mathematics is an important aspect of every child’s education. Although, I believe that its importance is lost throughout High School due to a lack of relative understanding of the concepts that students are being taught, meaning they are less likely to recognise links between concepts and overcome difficulties they encounter within maths problems (Skemp, 1989). This means that they are more likely to adopt a negative attitude to learning maths in the classroom. By giving our children space to be creative (see previous post titled ‘Maths can be… creative?’) and delve deeper into their understanding of different mathematical concepts, we are more likely to develop their awareness of the transferable nature of the skills that can be applied to different areas of maths and other wider connections. This leads to the development of ‘interconnectedness’ that Ma (2010) suggests is critical to having a profound understanding of mathematics, and thus recognising its importance in everyday life.

References

Ma, L (2010) Knowing and Teaching Elementary Mathematics. Oxon: Routledge.

Scottish Government (2012) Scottish Survey of Literacy and Numeracy 2011 (Numeracy). Available online at: http://www.scotland.gov.uk/Publications/2012/03/5285/downloads#res390565 [Accessed 3 October 2018].

Skemp, R. R. (1989) Mathematics in the Primary School. London: Routledge.

Maths can be… creative?

1 Reply

Creativity and mathematics are words which I did not associate with one another until recently. I believed that there was no room for creativity in mathematics as, after all, how can it be creative when it involves answering questions and problems which all have a ‘right’ answer? Oh how wrong I was.

Maths is all around us, from the parallel lines running through your carpet, to the tessellating tiles on your kitchen floor. It is not all about numbers, equations and ‘right’ answers, as shape, symmetry and proportion all come under the broad heading of mathematics. It is these areas which artists have been using for thousands of years to create beautiful patterns and images which form the basis of some of their artwork.

Islamic art in particular showcases how imaginative and creative maths can really be. Tessellation forms the basis of this type of artwork, allowing artists to create extraordinary repeat patterns from one simple shape.

One thing I find particularly interesting about tessellation is the ability to begin with one simple shape and transform it into another completely different one. Escher demonstrates this ability within his own work as a renowned graphic artist (see video of how he forms the basic unit of his patterns).

The video shows how something as simple as a hexagon can be transformed into a completely different shape altogether, just by chopping and changing parts of the shape (being a little creative with it!). The fact that this shape still tessellates even after being modified suggests the power of mathematics. Isn’t it amazing what we can achieve by experimenting and playing around with maths as we know it?

Price (2006) suggests that when we think of creativity, the expressive arts comes to mind due to our current curriculum restricting and confining what and how maths is taught within our schools. Should we, as teachers, be encouraging our students to experiment and take more risks within their maths – like Escher did – rather than teaching towards an objective? I believe that this would allow our children to see a different side to maths – like I have – rather than just the numbers, equations and ‘right’ answers that we (including myself) commonly think of when we hear the word maths.

As a student teacher now equipped with the knowledge of how important developing creativity within maths is for young children to be able to understand its purpose in society, I hope to provide my pupils with a range of opportunities within mathematics which will aid them in also recognising this. I aim to carry out professional reading about the teaching of maths in the primary school so that I can then demonstrate my own flexible understanding of maths with them, and thus become more confident in my own ability.

As, after all, why can’t maths be beautiful, creative and imaginative just like the expressive arts?

References

Giganti, P (2010) Anatomy of an Escher Lizard. Available at: https://www.youtube.com/watch?v=T6L6bE_bTMo&feature=youtu.be (Accessed: 26 September 2018).

Price, A. (2006) Creative Maths Activities for Able Students : Ideas for Working with Children Aged 11 to 14. London: Paul Chapman Publishing.

Scientific Literacy

Leave a reply

Recently within the primary school setting, teachers are finding it increasingly difficult to develop scientific literacy within children. This is due to the lack of understanding in the difference between scientific knowledge and scientific literacy. Throughout this essay we will focus on this explanation, and the effects of media reporting being inaccurate due to this lack of knowledge. We will also discuss fair testing in school and how this can be linked to scientific literacy.

In Maienschein’s (1998) analysis, she defines scientific literacy in two ways. Firstly, she describes ‘science literacy’ as the focus on specific scientific and/or technical knowledge which emphasises the knowledge of particular facts and skills. For example, being knowledgeable about factual information within science. This can often take over from the teaching of important life skills to children and focuses more on knowledge and facts that is often lost.
In her latter explanation, she focuses her attention on scientific processes and concepts whilst thinking critically and creatively about the natural world which in turn will help you to understand everyday life. For example, in a classroom situation we can explore and apply concepts such as gravity, using push/pull experiments. By carrying out lessons such as those discussed, teachers can encourage scientific literacy and ensure that learners deepen their understanding of these concepts. Using a hands-on approach will emphasise how the processes work and the importance of these in our lives. Furthermore, by developing scientific literacy, learners will be able to explore how they can live their best, most fulfilled lives.

A lack of scientific literacy is often linked to inaccurate media reporting, which can lead to the public gaining incorrect knowledge or understanding of a product. For example, in 2006, a ‘miracle cure’ for dyslexia appeared on the market. Wynford Dore claimed to have developed a drug-free dyslexia remedy and named it ‘Dore’.

The Mirror reported: “A revolutionary drug-free dyslexia remedy has been hailed a wonder cure by experts” (Mirror, 2012). However, a number of critical commentaries highlighted the flaws in the study behind the creation of Dore. Specifically, subjects were in fact not randomised and experimenters were able to place the child into either the control group or the treatment group which could have advantaged the Dore treatment.

Furthermore, the results of the treatment group were measured using screening tools instead of well validated tests which may have created biased results. The details of this treatment were withheld due to the fact it was “commercially sensitive”. The Independent reported that Dore used NASA technology and exercise in their remedy, which led to NASA making a public statement stating that they had nothing to do with the product, and that their technology was not used (Goldacre, 2006). The International Dyslexia Association (2004) stated that “interventions such as Mr. Dore’s are simply not supported by current knowledge.” The lack of scientific support for Dore’s dyslexia remedy may be one of the reasons that the Dore UK organisation went into administration and closed its centres in 2008 (Stephen Barrett, 2009).

Being able to conduct fair tests in school science is linked to the ability to use and understand scientific literacy. A fair test is one in which only one variable is changed, whilst the others are kept constant (Times Educational Supplement, 2015). This means that the test is reliable, and so valid conclusions can be drawn from it (Science Buddies, no date). Children can use the results from experiments to explore and understand the connections between the different variables being tested. The Office for Standards in Education (OFSTED) report titled ‘Maintaining Curiosity’ (2013) suggests that children best understand the concepts behind the science when they are actively involved in investigating them. The report highlighted that they learnt best when they could ‘see’ the science in action, which is what fair testing allows. Through this, fair testing can be seen as a strategy to deepen and encourage growth in children’s knowledge and understanding of scientific concepts.

References

Barrett, S. (2009) Dore Treatment Criticized. Available at: https://www.mentalhealthwatch.org/reports/dore2.shtml (Accessed: 8 Feb 2017)

Goldacre, B. (2006) Dore – The Miracle Cure for Dyslexia Available at: http://www.badscience.net/2006/11/the-miracle-cure-for-dyslexia/ (Accessed: 7 February 2017

Goldacre, B. (2008) ‘Determined bloggers who blew whistle’, The Guardian, 31 May. Available at: https://www.theguardian.com/commentisfree/2008/may/31/sciencenews.blogging (Accessed: 7 February 2017)

International Dyslexia Association (2004) Controversial Therapy (Dore Program) Lacks Research Basis. Available at: https://www.mentalhealthwatch.org/reports/dore.shtml (Accessed: 7 February 2017)

Maienschein, J, (1998) Scientific Literacy. Available at: http://science.sciencemag.org.libezproxy.dundee.ac.uk/content/281/5379/917 (Accessed: 6 Feb 2018).

Mirror (2012) ‘Dyslexia: The Cure’, 6 June. Available at: https://www.mirror.co.uk/news/uk-news/dyslexia-the-cure-647366 (Accessed: 7 February 2017).

Office for Standards in Education (2013) Maintaining Curiosity Available at: https://www.gov.uk/government/uploads/system/uploads/attachment_data/file/379164/Maintaining_20curiosity_20a_20survey_20into_20science_20education_20in_20schools.pdf (Accessed: 6 February 2017)

Science Buddies (no date) Variables for Beginners Available at: https://www.sciencebuddies.org/science-fair-projects/science-fair/doing-a-fair-test-variables-for-beginners (Accessed: 6 February 2017)

Times Educational Supplement (2015) The Fair Test (controlling variables) Available at: https://www.tes.com/teaching-resource/the-fair-test-controlling-variables-6060547 (Accessed: 6 February 2017).

Written by Katie Henderson, Carra Gulland, Chloe Newton and Lucy Somerville.

Critical Reflection on Semester 1

Leave a reply

Semester 1 was a whole new learning curve for me, especially working with other prospective students in the Social Work and Community Learning and Development courses for the ‘Working Together’ module.

Myself and seven others from the 3 professions came together to produce a presentation based on our visit to a Social Work agency and how well we thought we worked together as a group throughout the module. I was a bit timid to begin with in the group. I think this was because I came straight from school to University where I sat exams which assessed me purely on my own capabilities. This put me at a disadvantage when it came to collaborating within my group as it felt unusual to me to be challenged on my views as I had never really experienced this before, whereas others in my group had much more experience of working in a collaborative environment.

After some time in the group, I quickly began to contribute more to discussions as I saw the benefits of bringing everyone’s views together but also challenging them. I learnt to listen and take into consideration the views of others in my group and use it to develop my own views. This allowed us to come to varied and diverse answers and conclusions to different topics.

Moving forward, I will use this experience to engage with professions outside of the teaching community when on my Professional Practice, and to share my professional learning and development with colleagues as directed by section 3.4.2 of the Standards for Provisional Registration (The General Teaching Council for Scotland, 2012).

References:

The General Teaching Council for Scotland (2012). The Standards for Registration: mandatory requirements for Registration with the General Teaching Council for Scotland. Available at: http://www.gtcs.org.uk/web/FILES/the-standards/standards-for-registration-1212.pdf (Accessed: 19 January 2018).

A Poor View on Society

Leave a reply

After a recent lecture on Socio-Political Perspectives on Poverty, I realised how wrong I was about the ‘type’ of people who find themselves in the ‘lower class’ bracket of society.

http://www.bbc.co.uk/news/uk-32812601

Before going to the lecture, I was asked to write down my views on those who experience poverty; their characteristics, lifestyle, attitude to life etc. I found myself writing words such as ‘scrounger’, ‘homeless’, ‘stealing’, ‘unintellectual’ and the list went on. As I sat up from writing these words, I shocked myself about how easily these demeaning and negative attributes had come from the tip of my pen. I began to feel ashamed as I had always considered myself an open-minded person, able to form my own opinions, and thoughts about society after considering the perspectives of those within it. How had I come to such a harsh conclusion? Why did these terms appear on the paper without any second thought? Was this really my view of potentially the most vulnerable members of our society?

After considering my approach to the task for a while, I soon realised that actually, these were NOT my own views; these were my learned views. But what do I mean by ‘learned views’? I believe that another source has had such prominent views about poorer members of the community that I have unconsciously accepted or abided by these generalisations and stereotypes that they outwardly portray on a daily basis. But what is this incredibly influential source that has dominated my own personal thoughts and beliefs I hear you ask. It is something that is present even when I do not want to look at it, something that is alert even when I am not, something that preys on the weakest and most vulnerable members of society, and that something is… the media.

The media is notorious for producing ridiculous, one-sided headlines which primarily focus on people who ‘steal’ benefits which they are not entitled to, or are too lazy to go out and find ‘real jobs’. Headlines such as ‘Is Britain a nation of lazy scroungers?’ (2013)and ‘Mother-of-five who pockets £18,000 a year in benefits says she needs MORE handouts because she can’t afford to buy school uniforms for her children (but still manages to spend £20 a week on cigarettes)’ (2016) further the stereotypical view which I adopted in the task around people in poverty, devaluing those who are actually in need and are trying their best to provide for their family.

One particular video which I came across during my research into poverty was ‘I live in real poverty, and it’s not what you think’ in which Kathleen Kerridge illustrates the real life difficulties faced by her and her family in our modern day society.

Kathleen demonstrates how easily it is to fall into poverty without any warning. She challenges people like me who have followed the media’s views and have been coerced into believing that it is their own fault that they are in this situation, that she was to blame. She goes on to explain how none of the events which led to her poverty-stricken status were caused by her actions; her heart attack, losing her job, mastectomy and a bankrupt landlord. I think that this shows that this could happen to any of us, at any time, as she was once in a financially stable position.

Another video which I found which shocked me was that focussing on Shelby’s Story, titled ‘Teenage poverty in the UK’.

I was totally shocked when Shelby revealed she was 17 due to her living AND providing for herself. Being 17 myself, I could NEVER imagine coming home from my work (which I get paid over the minimum wage for) to my home with 2 rooms, no cooker and no bed. It made me realise that anyone can be a victim of poverty – poverty does not discriminate against age, gender or race.

I sat down again after researching Poverty in the UK and wrote down my revised perspective on poverty after seeing and hearing what poverty means to those who experience it daily. ‘Misunderstood’, ‘ordinary’ ‘unfortunate’ and ‘hard working’ were a few that I came up with, what I should of come up with to begin with.

As I sit typing on my laptop that I got for my 14th birthday, in my University flat, with my family and my dog in my 4 bedroom home 40 minutes away, I start to wonder what I will have for my tea. This is not because my cupboards are bare, or because I cannot afford to buy another meal like Kathleen or Shelby, but because I bought too much food when moving into my flat… and this is when I realised what real poverty is.

Poverty isn’t being ‘too lazy’ to go out and get a job. Poverty isn’t people who have spent their live scrounging off of the benefit system. Poverty is ordinary people. Ordinary people who have to count their pennies to see whether they can afford their next meal. Ordinary people who have to sleep on a broken bed because they cannot afford to replace it. Poverty is REAL and it is EVERYWHERE, and it most certainly should not be defined by what we see in the media.

Social Hierarchy – Resource Allocation Task

Leave a reply

As part of the first Values Workshop, we were split into 5 groups, each of which received an envelope containing stationary items. The aim of the task was to produce a pack, using the resources in your envelope, for new MA1 students. This would be given to them at the start of their University journey.

My group, Group 1, opened our envelope to see that we had a huge amount of stationary and craft items to use in order to make our pack. We decided to make a ‘Student Starter Guide’. This included the following:

a name badge;
a personalised timetable;
a map of the main University buildings;
tips aimed at the education course;
a leaflet of all the different social events/aspects of University;
and a guide to finding your way about the Dalhousie Building.

Derek was extremely enthusiastic towards our group, encouraging us every step of the way – he even offered us biscuits! We thrived off his energy and support for what we were making and felt proud of ur creative abilities. He kept us motivated and engaged in the task by continually checking in on our progress and praising our designs.

When it came to presenting our pack, it came as a great shock to me and the rest of my group to find out that Derek’s praise and the resources given to each group were not shared out equally. It turned out that, actually, this was planned! Some groups had as little as a few post-it notes, a pen and a paper clip in their envelope, whilst my group sat there with piles upon piles of unused paper and other resources. The encouragement each group received from Derek also varied depending on how many resources you had; the more resources you were given, the more praise you received. This was demonstrated right through to the very end of presenting the finished packs, as Derek paid very little attention to Groups 4 and 5 even when they were giving their talks – he even went on his phone during Group 5’s presentation!

We then went on to discuss what this task was really about in relation to the Teaching Profession. The fact that my group did not notice that other groups received less than us illustrates that pupils who come from a wealthy/higher class background and have access to a huge number of resources to aid their learning tend to show a degree of ignorance towards those who are from a lesser background. Also, those who were in the groups which received less equipment did not go to other groups to ask for more. This suggests that those who are from a lower class family and do not have many resources/services available to them feel that they cannot go out and ask for them. It was as if those in Groups 4 and 5 thought that they were not as deserving of the resources as those in Groups 1 and 2, hence why we need to make our pupils aware that no matter what background they come from, they are all equal in the classroom.

As teachers, we need to recognise that not everyone will come to school well-equipped with the skills, tools and support system needed to do well in their educational journey. It is our job to discourage these inequalities amongst children by pushing them to do their best despite the fact that their personal circumstances or social background might be seen as an obstacle which could potentially hold them back.

Another interesting point which was made was the fact that favouring those in your classroom from a higher class background can lead to them excelling and achieving far more than those from a lower class family. This can lead to pupils from deprived areas seeing themselves as ‘less’ than everyone else, therefore, they become unmotivated as they aren’t receiving the encouragement they need to aid success. A member of Group 5 even commented that she “did not see the point” in presenting their finished work as Derek seemed so disengaged in what they had to show, thus highlighting that unevenly distributing praise can have a huge impact on pupils’ motivation.

Overall, I learnt a huge amount during this task and it has opened my mind about the daily struggles we face in erasing the social hierarchy which has been embedded into our system. I thoroughly enjoyed the task and look forward to our next workshop!