What is Tessellation?

Tessellation is another word for tiling. Therefore, tessellation is when shapes are repeated over and over again covering a plane without any gaps or overlaps. There are different types of tessellation: regular, semi-regular and then other tessellations of circular, curved and irregular shapes that mathematicians agree on how to name.

Tessellation is used to look appealing to the eye, such as in art work or in peoples home, for example, tiles on walls or floors.

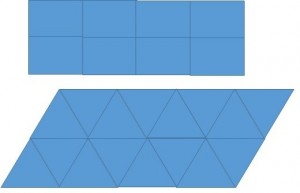
Below is a demonstration on how I tessellated squares and regular triangles.

Regular polygons in a tessellation must fill the plane at each vertex, the interior angle must be an exact divisor of 360 degrees. This works for the triangle, square, and hexagon. For all the others, the interior angles are not exact divisors of 360 degrees, and therefore those figures cannot tile the plane.

Where the corners meet, they will always add up to 360 degrees. The square has a 90 degree angle and 4 squares meet in one corner, which means that you can check mathematically that they will tessellate. 90 x 4 = 360. The same works for the equilateral triangle. Each angle on a triangle is 60 degrees. In the middle of the above diagram, 6 triangles meet at one corner. It is obvious from the diagram that they tessellate but we also know mathematically by doing 60 x 6 = 360.