In one of our Discovering Mathematics lectures we looked at the relationship between Maths and Art. I was interested to find out more about this link as it was not one that was immediately obvious to me.

There was a particular focus placed on the idea of the Golden Ratio.

The Golden Ratio can be represented as the Greek symbol phi:

http://media-cache-ak0.pinimg.com/736x/10/16/33/1016338c409d8a2bbb38f9555366e392.jpg

but is more commonly known as the ‘special’ number approximately equal to 1.618.

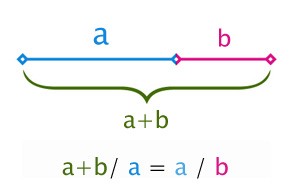
The theory behind the Golden Ratio is that when a line is divided into two parts, the longer section divided by the smaller section is equal to the full line divided by the longer section.

http://relativestrengthadvantage.com/ideal-proportions/diagram1.jpg

It is said that the Golden Ratio is very aesthetically pleasing, perhaps even beautiful, which may begin to explain the links between the underlying mathematic principles and art. This house emphasises how Maths can extend beyond the classroom and into the wider society as this is an example of the golden ration being used in architecture and design. The pillars and doors are of an equal length and the windows have all been carefully measured to ensure that they are of equal proportion, creating the perfect house.

http://cdn.freshome.com/wp-content/uploads/2014/09/classical-exterior.jpg

A coherent knowledge of how Maths can be linked to the wider society as well as other subjects means teachers can enable pupils to develop their skills in mathematics whilst also taking part in interdisciplinary learning as highlighted in the Curriculum for Excellence.

Have you ever come across a word, phrase or sequence that, when reflected, is exactly the same? Have you ever wondered what the term for this is? Well you are in luck because I can answer that. The name for this particular form is known as a palindrome. An example;

http://d33y93cfm0wb4z.cloudfront.net/ACTIVITIES_JO/WorksheetsForKids/PalinromesMAIN346x210.jpg

The phrase race car is a palindrome.

Can you guess this palindrome?

The phrase is taco cat.

I was both surprised and intrigued to find out that palindromes are not restricted to words, but as Rob Eastaway suggests in his book ‘How Many Socks Make A Pair?’ they can also be found in mathematics.

Take the multiplication sum:

3 x 7 x 11 x 13 x 37

The answer:

111,111

A perfect palindrome.

Eastaway (2010), identifies that the number 11 is not only featured in this pattern but also as the root number for numerous other aesthetically pleasing patterns. For example;

1 x 1 =                              1

11 x 11 =                       1 2 1

111 x 111 =                1 2 3 2 1

1111 x 1111 =         1 2 3 4 3 2 1

11111 x 11111 =  1 2 3 4 5 4 3 2 1

This palindrome has the ability to continue up until the number 111,111,111, however, this would require the solution to be acquired by hand as a standard calculator cannot display numbers over 12 digits long. Eastaway (2010) also suggests that there are many other forms of palindromes and pretty patterns that can be formed using numbers. Therefore, proving once again how mathematics can be linked to a variety of situations. Palindromes are traditionally looked within literacy however the links within mathematics prove to be very interesting as well.

References

Eastaway, R. (2010) How Many Socks Make A Pair? London: JR Books

“Why do we have numbers?”

At face value it looks like a simple enough question. However, i believe that there are too many reasons for why we have numbers to condense into one specific answer. So the question can simply be answered by looking at our life and realising that not a day goes by where we do not encounter numbers; whether we are telling the time; counting money; or setting an alarm (so that we can nap between lectures); the list is pretty endless. In order to familiarise myself with the terminology we were going to use during the lecture I decided to turn to google where I came across a website called www.dailywritingtips.com although perhaps not an educational website it did provide me with an article called “10 rules for writing numbers and writing numbers and numerals.” Michael the author suggested:

“A number is an abstract concept while a numeral is a symbol used to express the number.”

“One could say that the difference between a number and its numeral is like the difference between a person and her name.”

For me this short and sweet statement really cleared up the difference between the two terms.

During our lecture we took a look at the numerals we use;

ARABIC NUMERALS (also known as: European, Hindu, Hindu-Arabic, Indo-Arabic)

0 1 2 3 4 5 6 7 8 9 

However, there are other types of numerals in the world, such as;

ROMAN NUMERALS

1= I  2= II  3= III  4= IV  5= V  6= VI  7= VII  8= VIII 9= IX  10= X

CHINESE NUMERALS

http://prek-8.com/1stgrade/images/china6.jpg

MAYAN NUMERALS

http://s.hswstatic.com/gif/mayan-calender-5.jpg

We began to discussing the similarities and difference of each numerical system and soon it was time for us create a system of our own. We took into consideration the repetition used in Roman Numerals and decided to do something similar this time using triangles and when forming numbers above 9 we decided to apply the same rules as our own numerical system.

Once we had shared our creation with the other groups it was time to put them into an equation and it after a couple of attempts it surprisingly became like second nature and i found myself using it confidently.

The name we gave our numerical system was SLAREMUN – numerals backwards.