I personally believe that often in today’s world we can limit the idea of maths to calculations, equations and many hours of working things out. We don’t take time to consider just how complex and essential this subject is. Hom (2013) describes maths as “the science that deals with the logic of shape, quantity and arrangement”. It is not just something we do in a textbook to pass time, it can be applied to the real world and is the “building block” in all we do (Hom, 2013). It is all around us- in nature, music and photography.

Have you ever looked around at the beauty of creation and thought just how wonderful it is how everything comes together? How each hexagonal structure in honeycomb is so perfect and they all fit together? Or how symmetrical a butterflies wings are? How about the enormous amount of detail in a sunflower? A huge amount of maths is within this. I live near the Giant’s Causeway and have visited it too many times to count yet without fail every time I go I am always mesmerised by how the hexagonal rocks all fit together to form such a beautiful tourist spot. With Eddie, we looked at the art of a tessellation and the level of maths required to produce one. As the shapes need to fit perfectly together with no gaps or overlaps, you must consider the shapes you use e.g. you cannot use a pentagon by itself. The regular shapes that do tessellate are: squares, hexagons and equilateral triangles. All triangles and quadrilaterals also tile but they are not ‘regular’ shapes and you often have to rotate them to make them fit together. These shapes are, however, congruent, which means they are the same size. These congruent, irregular shapes make the monohedral tessellations (Valentine, 2017).

Tessellations of congruent shapes, such as above, are called monohedral tessellations. The word monohedral literally means ‘one’ – mono and ‘shape’ – hedral. Regular tessellations are made up of only one regular shape repeated, whilst semi-regular tessellations are made up of two or more regular shapes tiled to create a repeating pattern. A lot of Islamic art uses tessellations of equilateral triangles, squares and hexagons. Furthermore, in Spain there are many examples of art in tiling such Park Güell in Barcelona.

Interestingly, a family friend of mine is very involved with training teachers in mathematics and has created a course about learning mathematics through patchwork (Brown, 2017). I think this is an excellent idea. Not only is it creative and involves maths but is something that the children could make a mini version of to take home or make as an entire class for a display. This would be something for children to be proud of and they could feel a sense of achievement once completed. It would be a good cross-curricular link. I would consider this idea for an upper years class due to the materials required. It has inspired me to think of an activity for younger pupils where they can stick pieces of fabric onto paper to create their own tessellations.

Here is what my group came up with:

                           

The Fibonacci sequence has a huge part to play in the formation of sunflowers. This is a sequence made up of numbers where each number is determined by adding together the previous two numbers. For example- 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89 etc. Some scientists and keen beans on flowers have counted the seed spirals in a sunflower to confirm that it was indeed made up of the Fibonacci sequence. This is very common across a lot of plants and flowers and is actually why finding a four-leaf clover is considered so lucky as there are so few! Scientists believe that flowers form this way as it is the most efficient way to do so- they can “pack in the maximum number of seeds if each seed is separated by an irrational-numbered angle” such as Phi or the golden ratio (Life Facts, 2015). We looked into this a bit further with Anna Robb by dividing the length of our rectangles for the golden spiral by the width which came to a number very close to Phi (1.618…). The following video explains what we did in class (Graff, 2014).

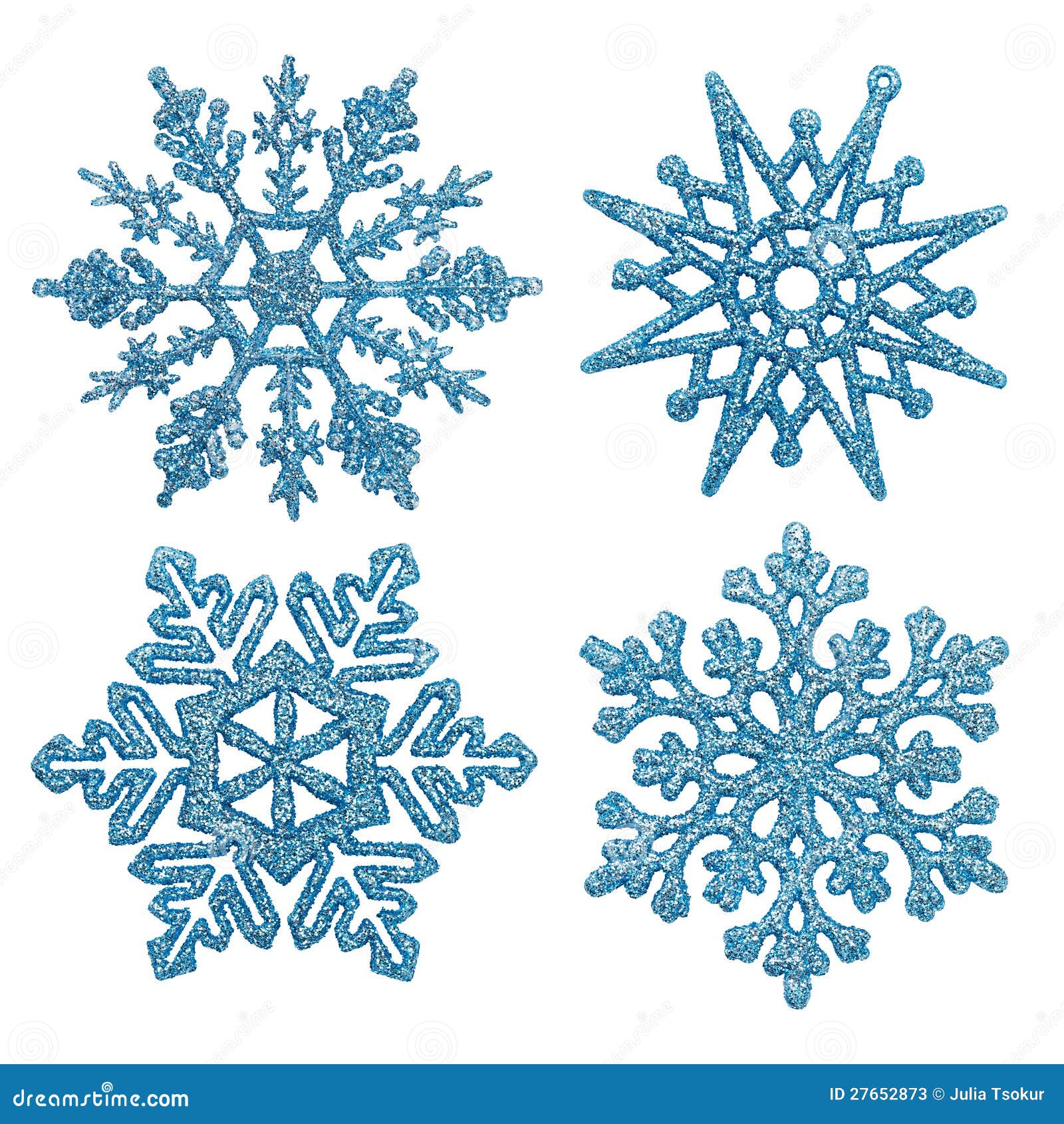Snowflakes are another example of maths in nature. They exhibit six-fold radial symmetry, with elaborate, identical patterns on each arm. Snowflakes are made entirely of water molecules which have solidified and crystallised to form weak hydrogen bonds with other water molecules. The bonds maximise attractive forces and reduce repulsive forces, allowing the snowflake to form its hexagonal shape (Life Facts, 2015). Isn’t it amazing how no two snowflakes are identical yet every snowflake is completely symmetrical? I wondered how this could happen and Life Facts (2015) gave me an answer- As no two snowflakes fall from the sky at the exact same time, they experience unique atmospheric conditions such as wind and humidity. This means that there is a different effect on every snowflake and how the crystals form. Each arm of the snowflake goes through identical conditions and therefore crystallises in the exact same way, resulting in a symmetrical snowflake.

During my placement in first year, I decided to do a lesson on how to draw a compass rose with Primary 6. This involved a lot of angle work to ensure that each point was at an equal angle to ensure the whole compass shape would work. It also involved consideration of the radius of circles and how to use a compass and ultimately the idea of direction. I found it quite a complicated lesson to teach as it required a high degree of accuracy which some of the children struggled with as many of them had not used a compass before. Furthermore, the whole class had only looked at using a protractor to measure and draw angles for the previous two lessons so lacked experience. I am, however, glad that I used this as a lesson as it was interesting and the children enjoyed the link between maths and art to produce their own compass. Here is the link to the process of drawing a compass rose (https://www.wikihow.com/Draw-a-Compass-Rose) and a photo of my final product.

Maths is even required in photography. Many photographers use the ‘Rule of Thirds’ to set up their photos. This is where the image in broken down into 9 sections using 4 lines. The idea is that if you capture an image where the main object/focus is placed along the lines or the intersections, the photo will be more natural and pleasing to the viewer instead at the centre of the shot (Rowse, no date). Another method photographers use is balancing elements. This is similar to the rule of thirds and is simply placing a focal point off centre to create a more interesting image, however, this means there is empty space at the opposite side. This is where balancing elements comes in- you place another similar object at the other side to balance the photo out- known as formal balance. Informal balance is when you place two varying objects at opposite sides of the image (Google, no date). Leading lines are another method used in photography in which straight objects such as roads are used to draw the viewer’s eye to the image and connect the foreground to the background (McKinnell, no date). The final method photographers use is symmetry and patterns within photos to create a balanced and aesthetically pleasing image (DMM, no date).

It is clear that maths is not just limited to textbooks, endless calculations and equations, it goes much further into the world of creative arts. I believe that more mathematical links need to be made within the classroom in subjects such as art to help child to explore all that the wonderful world of maths has to offer.

Brown, J. (2017) Learning Mathematics through Patchwork, 8 October 2016. Available at: https://www.linkedin.com/pulse/learning-mathematics-through-patchwork-jill-brown?trk=mp-reader-card (Accessed: 8 November 2017).

DMM (no date) How to Use Symmetry and Patterns in Photography. Available at: http://www.digimadmedia.com/blog-how-to-use-symmetry-and-patterns-in%20photography (Accessed: 4 November 2017).

Google (no date) Balancing Elements. Available at: https://sites.google.com/site/photographycompositionrules/balancing-elements (Accessed: 4 November 2017).

Graff, G. M. (2014) Understanding the Fibonacci Spiral. Available at: https://www.youtube.com/watch?v=8A3JnWzgXGk (Accessed: 4 November 2017).

Hom, E. J. (2013) What is Mathematics?. Available at: https://www.livescience.com/38936-mathematics.html (Accessed: 4 November 2017).

Life Facts (2015) 15 Beautiful Examples of Mathematics in Nature. Available at: http://www.planetdolan.com/15-beautiful-examples-of-mathematics-in-nature/ (Accessed: 4 November 2017).

McKinnell, A. (no date) How to Use Leading Lines for Better Compositions. Available at: https://digital-photography-school.com/how-to-use-leading-lines-for-better-compositions/ (Accessed: 4 November 2017).

Rowse, D. (no date) Rule of Thirds. Available at: https://digital-photography-school.com/rule-of-thirds/ (Accessed: 4 November 2017).

Valentine, E. (2017) Maths, creative? – No way! [PowerPoint Presentation], ED21006: Discovering Mathematics (year 2) (17/18). University of Dundee. 26 September.